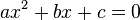This article is about single-variable quadratic equations and their solutions. For more general information about the single-variable case, see Quadratic function.

For the case of more than one variable, see Conic section or Quadratic form.

The quadratic formula for the roots of the general quadratic equation

In elementary algebra, a quadratic equation (from the Latin quadratus for "square") is any equation having the form

where x represents an unknown, and a, b, and c represent known numbers such that a is not equal to 0. If a = 0, then the equation is linear, not quadratic. The numbers a, b, and c are the coefficients of the equation, and may be distinguished by calling them, respectively, the quadratic coefficient, the linear coefficient and the constant or free term.^[1]

Because the quadratic equation involves only one unknown, it is called "univariate". The quadratic equation only contains powers of x that are non-negative integers, and therefore it is a polynomial equation, and in particular it is a second degree polynomial equation since the greatest power is two.

Source : https://en.wikipedia.org/wiki/Quadratic_equation

Atoms are the foundation of chemistry. They are the basis for everything in the Universe. As you know, matter is composed of atoms. Solids are made of densely packed atoms while gases have atoms that are spread out. We're going to cover basics like atomic structure and bonding between atoms. As you learn more, you can move to the reactions and biochemistry pages and see how atoms form compounds that help the biological world survive. 

Are there pieces of matter that are smaller than atoms? Sure there are. Super-small particles can be found inside the pieces of atoms. These subatomic particles include nucleons and quarks. Nuclear chemists and physicists work together at particle accelerators to discover the presence of these tiny, tiny, tiny pieces of matter. However, science is based on the atom because it is the smallest distinct unit of matter. 

Three Easy Pieces

Even though many super-tiny atomic particles exist, you only need to remember the three basic parts of an atom: electrons, protons, andneutrons. What are electrons, protons, and neutrons? Electrons are the smallest of the three particles that make up atoms. Electrons are found in shells ororbitals that surround the nucleus of an atom. Protons and neutrons are found in the nucleus. They group together in the center of the atom. That's all you have to remember. Three easy pieces! 

There are almost 120 known elements in the periodic table. (117 as we write this) Chemists and physicists are trying to make new ones every day in their labs. The atoms of different elements have different numbers of electrons, protons, and neutrons. Every element is unique and has an atomic number. That number tells you the number of protons in every atom of the element. The atomic number is also called the proton number. 

Charges of Atoms

You can see that each part of the atom is labeled with a "+", "-", or a "0." Those symbols refer to the charge of the particle. Have you ever heard about getting a shock from a socket, static electricity, or lightning? Those are all related to electric charges. Charges are also found in tiny particles of matter. 

The electron always has a "-", or negative, charge. The proton always has a "+", or positive, charge. If the charge of an entire atom is "0", or neutral, there are equal numbers of positive and negative charges. Neutral atoms have equal numbers of electrons and protons. The third particle is the neutron. It has a neutral charge, also known as a charge of zero. 

Since the number of protons in an atom does not change, fewer or extra electrons can create a special atom called an ion. Cations have fewer electrons and have a positive charge. Anions have extra electrons that create a negative charge. 


Source : http://www.chem4kids.com/files/atom_structure.html

The notion of line or straight line was introduced by ancient mathematicians to represent straight objects (i.e., having no curvature) with negligible width and depth. Lines are an idealization of such objects. Until the 17th century, lines were defined in this manner: "The [straight or curved] line is the first species of quantity, which has only one dimension, namely length, without any width nor depth, and is nothing else than the flow or run of the point which […] will leave from its imaginary moving some vestige in length, exempt of any width. […] The straight line is that which is equally extended between its points"^[1]

Euclid described a line as "breadthless length" which "lies equally with respect to the points on itself"; he introduced several postulates as basic unprovable properties from which he constructed the geometry, which is now called Euclidean geometry to avoid confusion with other geometries which have been introduced since the end of 19th century (such as non-Euclidean, projective and affine geometry).

In modern mathematics, given the multitude of geometries, the concept of a line is closely tied to the way the geometry is described. For instance, in analytic geometry, a line in the plane is often defined as the set of points whose coordinates satisfy a given linear equation, but in a more abstract setting, such as incidence geometry, a line may be an independent object, distinct from the set of points which lie on it.

When a geometry is described by a set of axioms, the notion of a line is usually left undefined (a so-called primitive object). The properties of lines are then determined by the axioms which refer to them. One advantage to this approach is the flexibility it gives to users of the geometry. Thus in differential geometry a line may be interpreted as a geodesic (shortest path between points), while in some projective geometriesa line is a 2-dimensional vector space (all linear combinations of two independent vectors). This flexibility also extends beyond mathematics and, for example, permits physicists to think of the path of a light ray as being a line.

A line segment is a part of a line that is bounded by two distinct end points and contains every point on the line between its end points. Depending on how the line segment is defined, either of the two end points may or may not be part of the line segment. Two or more line segments may have some of the same relationships as lines, such as being parallel, intersecting, or skew, but unlike lines they may be none of these, if they are coplanar and either do not intersect or are collinear.

Source : https://en.wikipedia.org/wiki/Line_(geometry)

Electrostatics is a branch of physics that deals with the phenomena and properties of stationary or slow-moving electric charges with no acceleration.

Since classical physics, it has been known that some materials such as amber attract lightweight particles after rubbing. The Greek word for amber, ήλεκτρον electron, was the source of the word 'electricity'. Electrostatic phenomena arise from the forces that electric charges exert on each other. Such forces are described by Coulomb's law. Even though electrostatically induced forces seem to be rather weak, the electrostatic force between e.g. an electron and a proton, that together make up a hydrogen atom, is about 36 orders of magnitude stronger than the gravitational force acting between them.

There are many examples of electrostatic phenomena, from those as simple as the attraction of the plastic wrap to your hand after you remove it from a package, and the attraction of paper to a charged scale, to the apparently spontaneous explosion of grain silos, the damage of electronic components during manufacturing, and the operation of photocopiers. Electrostatics involves the buildup of charge on the surface of objects due to contact with other surfaces. Although charge exchange happens whenever any two surfaces contact and separate, the effects of charge exchange are usually only noticed when at least one of the surfaces has a highresistance to electrical flow. This is because the charges that transfer to or from the highly resistive surface are more or less trapped there for a long enough time for their effects to be observed. These charges then remain on the object until they either bleed off to ground or are quickly neutralized by a discharge: e.g., the familiar phenomenon of a static 'shock' is caused by the neutralization of charge built up in the body from contact with insulated surfaces.